Physicists have been able to test in condensed matter the prediction of Schwinger (1951) concerning the instability of vacuum with respect to the creation of particle-antiparticle pairs under strong electric field. This result obtained in dimension d=1, confirms the validity of non-perturbative approaches, and promotes graphene for a laboratory quantum electrodynamics (QED).
One of the most striking predictions of quantum field theory is the instability of an electric field upon creation of particle-antiparticle pairs, known as the Schwinger effect. The rate of creation, which depends on the dimensionality, has a threshold electric field determined by the mass of the particles and the speed of light. This is where the problem lies, since fields of 1018 V⁄m would be needed to create electron-positron pairs of energy 511 keV. Such fields are inaccessible today, except perhaps one day at the convergence of ultra-powerful laser beams where its observation is one of the grails. In the meantime, condensed matter analogues can be useful in simulating these “high energy” mechanisms in the laboratory to test the theories behind them.
The interest in graphene for QED was foreseen by the graphene pioneers. It is due to the relativistic character of the elementary excitations of the electron and hole type, with a speed of light replaced by the Fermi speed which is 300 times lower, a chirality and an ambipolarity which make them true Dirac fermions. Some relativistic aspects of transport in graphene have been well studied such as the Klein tunneling effect which leads to a collimation of electrons in a p-n junction, but the Schwinger effect remained elusive. The reasons for this are both technical and conceptual: on the one hand, high quality graphene was needed to realize ballistic devices on large dimensions, and on the other hand, it was necessary to understand the electronic transport in these strong field regimes where the electrons are extremely out of equilibrium. These advances allowed the experiment published in the on-line version of March 9th of Nature Physics, which was performed by the Mesoscopic Physics, Nano-Optics, and Fundamental Interactions teams of LPENS, in collaboration with theorists from LOMA-Bordeaux and LPS-Orsay.
The key came from long transistors based on graphene encapsulated in boron nitride, showing a characteristic exponential current saturation at high voltage. The saturation is induced by the strong electric field concentrated on a length of the order of a micron at the drain of the transistor in the pinch-off regime. This pinchoff effect is well known and even crucial for the operation of semiconductor transistors in our computers. For semi-metallic graphene the pinchoff is stranger and has in fact a very singular character specific to Dirac fermions: the applied electric field induces a giant Klein collimation of the electrons leading to a one-dimensional transport with an exponential saturation of the current. The paper shows that the collimation field is ultimately limited by the Schwinger instability of electron-hole pair creation. The associated Schwinger conductance has a remarkable universal form in d=1 dimension, which is measured in quantitative agreement with the theory in its non-perturbative version. This doubly relativistic variant, where the Schwinger effect arises from the Klein collimation, has been called the mesoscopic Klein-Schwinger effect.
The observations reported in the paper, constitute an important advance in the understanding of the intrinsic limits to current saturation in graphene, and open the way for new laboratory QED experiments.
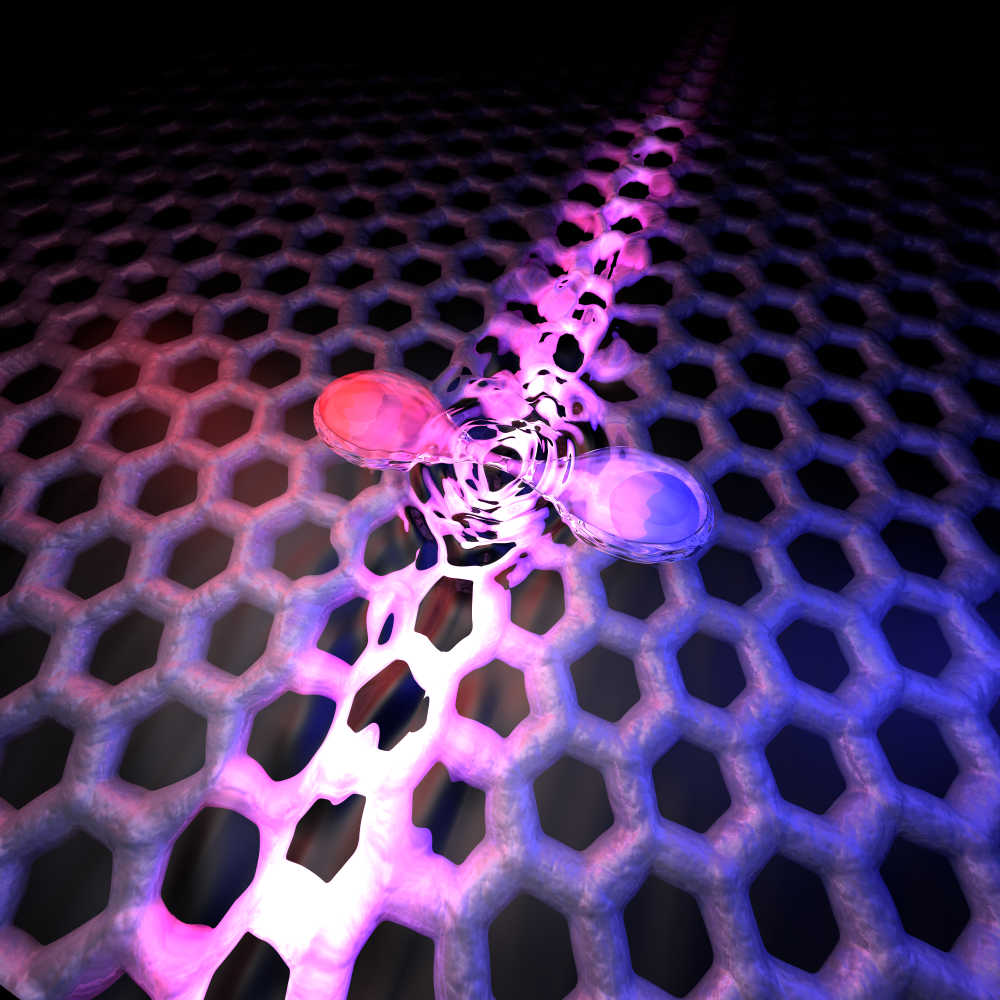
Artist’s view of vacuum polarization by electron-hole pair creation in graphene.
More:
https://doi.org/10.1038/s41567-023-01978-9
Aurthor affiliation:
Laboratoire de physique de L’École normale supérieure (LPENS, ENS Paris/CNRS/Sorbonne Université/Université de Paris)
Corresponding author: Bernard Plaçais – Emmanuel Baudin – Jan Troost
Communication contact: L’équipe de communication